Investigation: b_1≠0,b_5=0

We reduce the scalar product: By applying a Lie-algebra automorphism, we yield the implication b_1≠0⇒b_4=0.

subst = {b_3→0, b_4→0, b_5→0} ;

Τα[B/.subst, 0, EXP[λ M[[4]]]/.λ→λ]//MF

( {{b_1, b_2, 0, λ b_2}, {b_2, 0, b_6, b_7}, {0, b_6, b_8, λ b_6 + b_9}, {λ b_2, b_7, λ b_6 + b_9, 2 λ b_7 + b_10}} )

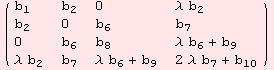
We compute the geometric tensors:

eqs = ShowGeo[{b_3→0, b_4→0, b_5→0}] ;

We consider the scalar product ℬ = ( {{b_1, b_2, 0, 0}, {b_2, 0, b_6, b_7}, {0, b_6, b_8, b_9}, {0, b_7, b_9, b_10}} )

with determinant<br /> |ℬ| = -b_1 (b_7^2 b_8 - 2 b_6 b_7 b_9 + b_6^2 b_10) + b_2^2 (b_9^2 - b_8 b_10)

The conditions det[B]≠0, and Ric=0 imply

Reduce[eqs]

False

Created by Mathematica  (September 15, 2007) Valid XHTML 1.1!