Structure equation of Mauer-Catan form
We continue to work with the Lie group ![]() R and its familiar commutator relation.
R and its familiar commutator relation.
![]()
![]()
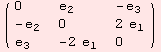
In the section on left-invariant tensor fields, we have made implicit use of the following form ![]() :X(G)→g, called Mauer-Catan form. The function of the kernel to compute the form, however, is denoted GdL. This is because
:X(G)→g, called Mauer-Catan form. The function of the kernel to compute the form, however, is denoted GdL. This is because ![]() =
=![]()
![]() .
.
![]()
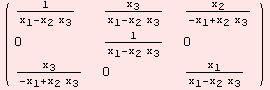
We would like to check the structure equation dω(X,Y)=-![]() [ω(X),ω(Y)]=-
[ω(X),ω(Y)]=-![]() ad.ω.X.ω.Y for all vector fields X,Y∈X(G). The bold "d" denotes the differential on forms. First, we determine dω.
ad.ω.X.ω.Y for all vector fields X,Y∈X(G). The bold "d" denotes the differential on forms. First, we determine dω.
![]()
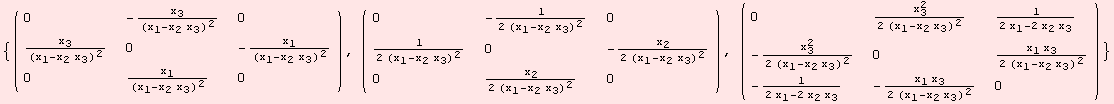
The next result corresponds to -![]() ad.ω.ω. The two tensors fields are equal.
ad.ω.ω. The two tensors fields are equal.
![]()

| Created by Mathematica (September 30, 2006) |