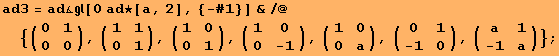
All real 3-dimensional Lie algebras
The following list was taken from the paper "Invariants of real low dimension Lie algebras" [Patera et al.]. Algebraic sums of lower dimensional Lie algebras are omitted
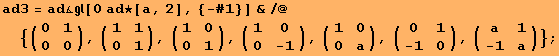
![AppendTo[ad3, Table2ad[({{0, e_1, -2e_2}, {0, 0, e_3}, {0, 0, 0}})]] ;](../HTMLFiles/index_387.gif)
![AppendTo[ad3, Table2ad[({{0, e_3, -e_2}, {0, 0, e_1}, {0, 0, 0}})]] ;](../HTMLFiles/index_388.gif)
The following list summarizes the commutator tables. The basis is 
,
,
. The coefficient a ranges over special intervals. First 0<a<1, secondly 0<a.
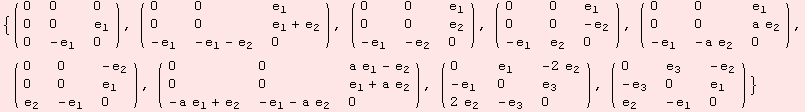
The killing form reveals, which of the algebras are solvable, and which are semi-simple.
![ShowMat[adKilling/@ad3]](../HTMLFiles/index_394.gif){kind=small}
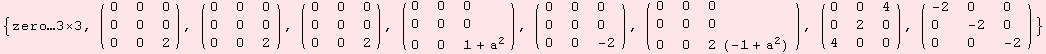
According to a Theorem, κ([g,g],g)=0 is equivalent to solvability. The first 7 algebras are solvable. We confirm for instance
![ = NS[NS[Join @@ T[ad = ad3[[7]], {3, 1, 2}]]] ;](../HTMLFiles/index_396.gif){kind=small}
![E0[ . adKilling[ad]]](../HTMLFiles/index_397.gif){kind=small}
The characteristic polynomials with roots of the 7 algebras are
![{χ = adCharacter[#1, x, λ], Solve[E0[χ], λ]} &/@ad3//MF](../HTMLFiles/index_399.gif){kind=small}
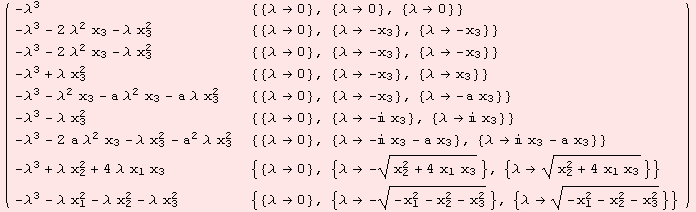
The chapter on Lie groups, we derive Lie group actions that induce all algebras listed here. 
is an exception.
| Created by Mathematica (September 30, 2006) |  |